André Joyal
André Joyal is a professor of mathematics at the Université du Québec à Montréal who works on category theory. Joyal was born in Drummondville (formerly Saint-Majorique). He has three children and lives in Montreal.
Contents |
Main research
He invented Kripke–Joyal semantics,[1] the theory of combinatorial species and with M. Tierney a generalization of the Galois theory of Grothendieck[2] in the setup of locales. Most of his research is in some way related to category theory, higher category theory and their applications. He did the first real work on quasi-categories, after their invention by Boardman and Vogt, in particular conjecturing.[3] and proving the existence of a Quillen model structure on sSet whose weak equivalences generalize both equivalence of categories and Kan equivalence of spaces. He co-authored the book "Algebraic Set Theory" with Ieke Moerdijk and recently started a web-based expositional project Joyal's CatLab [4] on categorical mathematics.
Works on algebraic equations
Joyal proved the following theorem in 1967.
If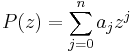 
is a polynomial of degree n such that 
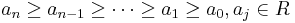, then all the zeros of P(z) lie in 
.[5]
References
- ^ Robert Goldblatt, A Kripke-Joyal semantics for noncommutative logic in quantales; Advances in Modal Logic 6, 209--225, Coll. Publ., London, 2006; MR2008m:03047
- ^ A. Joyal, M. Tierney, An extension of the Galois theory of Grothendieck, Mem. Amer. Math. Soc. 51 (1984), no. 309, vii+71 pp.
- ^ A. Joyal, A letter to Grothendieck, April 1983 (contains a Quillen model structure on simplicial presheaves)
- ^ Joyal's CatLab
- ^ A.Joyal, G. Labelle and Q.I.Rehman, On the location of zeros of polynomial, Canad. Math. Bull. 10, (1967), 53–63, MR0213513
- A. Joyal, Quasi-categories and Kan complexes, (in Special volume celebrating the 70th birthday of Prof. Max Kelly) J. Pure Appl. Algebra 175 (2002), no. 1-3, 207—222 doi.
- A. Joyal, M. Tierney, Quasi-categories vs Segal spaces, Categories in algebra, geometry and mathematical physics, 277—326, Contemp. Math. 431, Amer. Math. Soc., Providence, RI, 2007. math.AT/0607820.
- A. Joyal, M. Tierney, On the theory of path groupoids, J. Pure Appl. Algebra 149 (2000), no. 1, 69—100, doi
- A. Joyal, R. Street, Pullbacks equivalent to pseudopullbacks, Cahiers topologie et géométrie différentielle catégoriques 34 (1993) 153-156; numdam MR94a:18004.
- A. Joyal, M. Tierney, Strong stacks and classifying space, Category theory (Como, 1990), 213—236, Lecture Notes in Math. 1488, Springer 1991.
- A. Joyal, Ross Street, An introduction to Tannaka duality and quantum groups, Category theory (Como, 1990), 413—492, Lecture Notes in Math. 1488, Springer 1991 pdf.
- A. Joyal, R. Street, The geometry of tensor calculus I, Adv. Math. 88(1991), no. 1, 55—112, doi; Tortile Yang-Baxter operators in tensor categories, J. Pure Appl. Algebra 71 (1991), no. 1, 43—51, doi; Braided tensor categories, Adv. Math. 102 (1993), no. 1, 20—78, doi.
- A. Joyal, R. Street, D. Verity, Traced monoidal categories. Math. Proc. Cambridge Philos. Soc. 119 (1996), no. 3, 447—468.
- A. Joyal, I. Moerdijk, Algebraic set theory. London Mathematical Society Lecture Note Series 220. Cambridge Univ. Press 1995. viii+123 pp. ISBN 0-521-55830-1
- A. Joyal, The theory of quasi-categories and its applications, lectures at CRM Barcelona February 2008, draft pdf
- A Joyal, Notes on quasicategories, draft pdf
- A. Joyal, M. Tierney, Notes on simplicial homotopy theory, CRM Barcelona, Jan 2008 pdf
- A. Joyal, Disks, duality and theta-categories, preprint (1997) (contains an original definition of a weak $n$-category: for a short account see Leinster's book, 10.2).